Research of the Posch Group
Research of the Posch Group
Lyapunov Instability of Many-Body Systems
The phase-space trajectory of a many-body system consisting of atoms or molecules is highly Lyapunov unstable: due to the convex surface of the particles, a small perturbation of the initial conditions typically grows, or shrinks, exponentially with time. The rate of this growth/decay is characterized by a set of Lyapunov exponents, the so-called Lyapunov spectrum. The Lyapunov instability is ultimately responsible for the statistical properties of matter. Two recent discoveries of our group have raised much interest:(a) The perturbation associated with the most-unstable directions in phase space and contributing to the maximum Lyapunov exponent is strongly localized in space. This localization persists in the thermodynamic limit of infinitely-many particles.(b) For hard-disk and hard-sphere systems the perturbations belonging to the smallest of the nonvanishing exponents are coherently spread out in space, reminiscent of the classical modes of fluctuating hydrodynamics. We refer to these periodic structures as "Lyapunov modes". There exist non-propagating "transversal" modes, and propagating "longitudinal" modes. We have characterized the Lyapunov modes and have extended these considerations to large systems close to the thermodynamic limit. Our results have sparked considerable theoretical activity in various groups around the world.
Chaos and Irreversibility
In 1986 Professor William G. Hoover, Dr. Brad L. Holian and I discovered that the phase-space probability density of dynamically-thermostated stationary nonequilibrium systems collapses onto a fractal attractor. The dimension of such a fractal is smaller than the dimension of the equilibrium phase space. The dimensionality loss is related to the rate of irreversible entropy production, and is a consequence of the Second Law of thermodynamics. It explains, for example, why such systems have an "arrow of time" and behave irreversibly in spite of their time-reversible equations of motion.
To generate a nonequilibrium flow, an external perturbation is needed. It drives the system away from equilibrium. However, such a perturbation performs work on the system and would raise the temperature without a suitable thermostat or ergostat. It removes the excessive heat and establishes a stationary state. However, the time-reversible thermostats, which we used in 1986 and which are currently the standard procedure, have led to an interesting controversy in the literature questioning their physical significance. Recently, we have been able to show that the dimensionality loss mentioned above may exceed by far the dimensions contributed by the thermostatted degrees of freedom for a system far from equilibrium.
We have also demonstrated that it is possible to replace the time-reversible dynamical thermostats by stochastic thermostats and to construct Lyapunov spectra for such systems, which share the desired properties with their time-reversible counterparts. This shows that dynamical thermostats are not merely convenient numerical tools but may be a first stepping stone towards a theory of nonequilibrium stationary states.
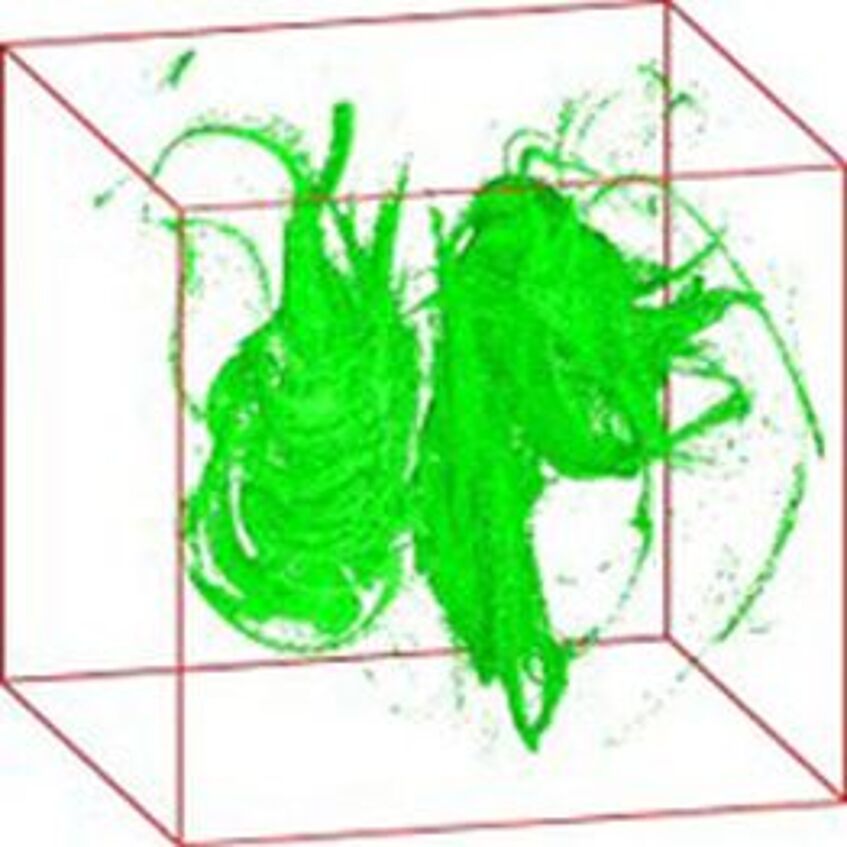
Gravitating Systems
The asymptotic states of gravitational many-body systems confined to a box depend on the number of effective constants of the motion. With reflecting boundaries (a scenario reminiscent of a star cluster caught in the self-consistent field of a galaxy), the existence of an additional conserved quantity other than the energy determines whether three bodies may reach a finite stationary state or will collapse forever. Our recent work clarifies some of the open questions and, as usual, provides an interesting new puzzle concerning unexpected vanishing Lyapunov exponents and, hence, hitherto unknown constraints in phase space.
Systems with Negative Specific Heat
Recent experiments on nuclear fragmentation and atomic clusters indicate an unusual feature, namely a negative specific heat. This seems to contradict a general result of Lebowitz and Lieb according to which a Coulomb system of electrons and nuclei always has a positive specific heat, even in the microcanonical ensemble. This theorem presupposes ergodicity and the thermodynamic limit. We have recently demonstrated that the non-compliance of any of these requirements by an experiment provides a possible pathway to a negative specific heat.
Structure and Dynamics of Liquid Mixtures
In a thorough experimental study of a liquid mixture, benzonitrile - toluene, we have carried outi) a number of thermodynamic measurements,ii) Rayleigh and Raman light-scattering spectroscopy, iii) NMR 13-C spin-lattice relaxation measurements, andiv) measurements of the cross correlation between dipolar nuclear spin relaxation and chemical shift anisotropy.
Combining these data with computer simulations, we obtain one of the most-comprehensive pictures of the molecular structure and the reorientational dynamics of any liquid mixture available today.
